Tutorial: Conical Horn Antenna

From openEMS

Download the latest matlab file using Github: Conical_Horn_Antenna.m
Simulation Time: < 10 min
Far-field calculations: ~ 1 hour

This tutorial covers:

setup conical horn antenna using a rotated polygon
setup a rectangular feeding port
adding a near-field to far-field (nf2ff) box
calculate the S-Parameter of the antenna
calculate and plot the far-field pattern

Matlab Simulation Script

Start the script within an empty environment:

close all
clear
clc

Setup the simulation parameters

physical_constants;
unit = 1e-3; % all length in mm

Specify the conical horn model parameters

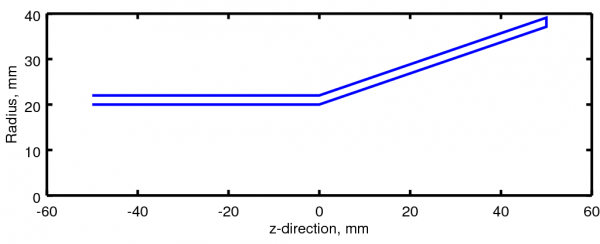
% horn radius
horn.radius  = 20; 
% horn length in z-direction
horn.length = 50;
horn.feed_length = 50; 
horn.thickness = 2;
 
% horn opening angle
horn.angle = 20*pi/180;

Note that horn.radius is actually the radius of the circular waveguide which feeds the horn.

The parameter horn.feed_length is the length of the circular waveguide.

The thickness of the metal which forms the horn is set by horn.thickness.

Specify the simulation parameters

% size of the simulation box
SimBox = [100 100 100]*2;
 
% frequency range of interest
f_start =  10e9;
f_stop  =  20e9;
 
% frequency of interest
f0 = 15e9;
 
%% setup FDTD parameter & excitation function
FDTD = InitFDTD( 'NrTS', 30000, 'EndCriteria', 1e-4 );
FDTD = SetGaussExcite(FDTD,0.5*(f_start+f_stop),0.5*(f_stop-f_start));
BC = {'PML_8' 'PML_8' 'PML_8' 'PML_8' 'PML_8' 'PML_8'}; % boundary conditions
FDTD = SetBoundaryCond( FDTD, BC );

Define the mesh

%% setup CSXCAD geometry & mesh
% currently, openEMS cannot automatically generate a mesh
max_res = c0 / (f_stop) / unit / 15; % cell size: lambda/20
CSX = InitCSX();
 
%create fixed lines for the simulation box, substrate and port
mesh.x = [-SimBox(1)/2 -horn.radius 0 horn.radius SimBox(1)/2];
mesh.x = SmoothMeshLines( mesh.x, max_res, 1.4); % create a smooth mesh between specified fixed mesh lines
 
mesh.y = mesh.x;
 
%create fixed lines for the simulation box and given number of lines inside the substrate
mesh.z = [-horn.feed_length 0 SimBox(3) ];
mesh.z = SmoothMeshLines( mesh.z, max_res, 1.4 );
 
CSX = DefineRectGrid( CSX, unit, mesh );

Note that the mesh in the z-direction does not cover the range set by SimBox: the mesh starts at -horn.feed_length and goes to +200.

Even though the horn is rotationally symmetric, a rectangular grid is still used.

Image of the model mesh in the x-z plane.

Specify the geometry of the horn and the waveguide feed

%% create horn
% horn + waveguide, defined by a rotational polygon
CSX = AddMetal(CSX, 'Conical_Horn');
p(1,1) = horn.radius+horn.thickness;   % x-coord point 1
p(2,1) = -horn.feed_length;     % z-coord point 1
p(1,end+1) = horn.radius+horn.thickness;   % x-coord point 1
p(2,end) = 0;     % z-coord point 1
p(1,end+1) = horn.radius+horn.thickness + sin(horn.angle)*horn.length; % x-coord point 2
p(2,end) = horn.length; % y-coord point 2
p(1,end+1) = horn.radius + sin(horn.angle)*horn.length; % x-coord point 2
p(2,end) = horn.length; % y-coord point 2
p(1,end+1) = horn.radius;  % x-coord point 1
p(2,end) = 0;     % z-coord point 1
p(1,end+1) = horn.radius;   % x-coord point 1
p(2,end) = -horn.feed_length;     % z-coord point 1
CSX = AddRotPoly(CSX,'Conical_Horn',10,'x',p,'z');
 
% horn aperture
A = pi*((horn.radius + sin(horn.angle)*horn.length)*unit)^2;

Polygon which is rotated about the z-axis to generate the conical horn model.

Define the excitation

%% apply the excitation %%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%
start=[-horn.radius -horn.radius mesh.z(10) ];
stop =[+horn.radius +horn.radius mesh.z(1)+horn.feed_length/2 ];
[CSX, port] = AddCircWaveGuidePort( CSX, 0, 1, start, stop, horn.radius*unit, 'TE11', 0, 1);

The circular waveguide port runs from z = -41.176 to -25.

Set up field storage and run simulation

CSX = AddDump(CSX,'Exc_dump');
start=[-horn.radius -horn.radius mesh.z(8)];
stop =[+horn.radius +horn.radius mesh.z(8)];
CSX = AddBox(CSX,'Exc_dump',0,start,stop);
 
%% nf2ff calc
start = [mesh.x(9) mesh.y(9) mesh.z(9)];
stop  = [mesh.x(end-8) mesh.y(end-8) mesh.z(end-8)];
[CSX nf2ff] = CreateNF2FFBox(CSX, 'nf2ff', start, stop, 'Directions', [1 1 1 1 0 1]);
 
%% prepare simulation folder
Sim_Path = 'tmp';
Sim_CSX = 'horn_ant.xml';
 
[status, message, messageid] = rmdir( Sim_Path, 's' ); % clear previous directory
[status, message, messageid] = mkdir( Sim_Path ); % create empty simulation folder
 
%% write openEMS compatible xml-file
WriteOpenEMS( [Sim_Path '/' Sim_CSX], FDTD, CSX );
 
%% show the structure
CSXGeomPlot( [Sim_Path '/' Sim_CSX] );
 
%% run openEMS
RunOpenEMS( Sim_Path, Sim_CSX);

Note in the CreateNF2FFBox command that the -z direction has been disabled because the model touches the -z boundary of the simulation space.

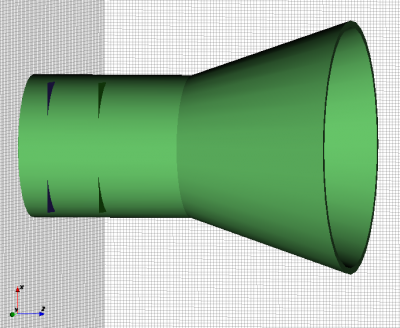
Model of the conical horn. The corners of the planes which define the waveguide port can be seen protruding from the circular waveguide.

Gridded model of the conical horn.

Postprocessing

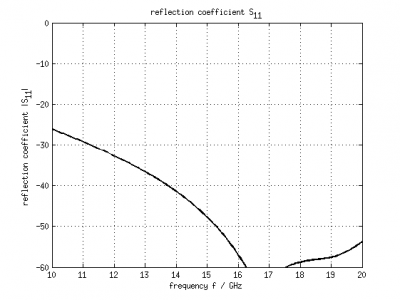
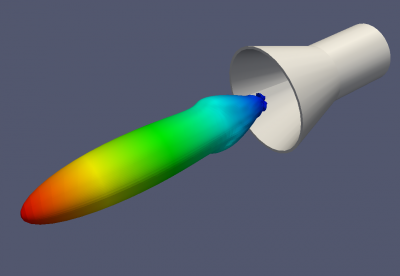
%% postprocessing & do the plots
freq = linspace(f_start,f_stop,201);
 
port = calcPort(port, Sim_Path, freq);
 
Zin = port.uf.tot ./ port.if.tot;
s11 = port.uf.ref ./ port.uf.inc;
 
% plot reflection coefficient S11
figure
plot( freq/1e9, 20*log10(abs(s11)), 'k-', 'Linewidth', 2 );
ylim([-60 0]);
grid on
title( 'reflection coefficient S_{11}' );
xlabel( 'frequency f / GHz' );
ylabel( 'reflection coefficient |S_{11}|' );
 
drawnow
 
%% NFFF contour plots %%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%
 
% calculate the far field at phi=0 degrees and at phi=90 degrees
thetaRange = (0:2:359) - 180;
disp( 'calculating far field at phi=[0 90] deg...' );
nf2ff = CalcNF2FF(nf2ff, Sim_Path, f0, thetaRange*pi/180, [0 90]*pi/180);
 
Dlog=10*log10(nf2ff.Dmax);
G_a = 4*pi*A/(c0/f0)^2;
e_a = nf2ff.Dmax/G_a;
 
% display some antenna parameter
disp( ['radiated power: Prad = ' num2str(nf2ff.Prad) ' Watt']);
disp( ['directivity: Dmax = ' num2str(Dlog) ' dBi'] );
disp( ['aperture efficiency: e_a = ' num2str(e_a*100) '%'] );
 
%%
% normalized directivity
figure
plotFFdB(nf2ff,'xaxis','theta','param',[1 2]);
drawnow
%   D_log = 20*log10(nf2ff.E_norm{1}/max(max(nf2ff.E_norm{1})));
%   D_log = D_log + 10*log10(nf2ff.Dmax);
%   plot( nf2ff.theta, D_log(:,1) ,'k-', nf2ff.theta, D_log(:,2) ,'r-' );
 
% polar plot
figure
polarFF(nf2ff,'xaxis','theta','param',[1 2],'logscale',[-40 20], 'xtics', 12);
drawnow
%   polar( nf2ff.theta, nf2ff.E_norm{1}(:,1) )
 
%% calculate 3D pattern
phiRange = sort( unique( [-180:5:-100 -100:2.5:-50 -50:1:50 50:2.5:100 100:5:180] ) );
thetaRange = sort( unique([ 0:1:50 50:2.:100 100:5:180 ]));
 
disp( 'calculating 3D far field...' );
nf2ff = CalcNF2FF(nf2ff, Sim_Path, f0, thetaRange*pi/180, phiRange*pi/180, 'Verbose',2,'Outfile','nf2ff_3D.h5');
 
figure
plotFF3D(nf2ff);        % plot liear 3D far field
 
%%
E_far_normalized = nf2ff.E_norm{1}/max(nf2ff.E_norm{1}(:));
DumpFF2VTK([Sim_Path '/Conical_Horn_Pattern.vtk'],E_far_normalized,thetaRange,phiRange,'scale',1e-3);

Results

Antenna return loss

Antenna structure and radiation pattern

Tutorial: Conical Horn Antenna

From openEMS

Contents

This tutorial covers:

Matlab Simulation Script

Results

Return To Tutorials Index

Navigation

documentation